Gravitational attraction and the planets

If you were to make a journey across our Solar System and land on different planets you would notice that you weighed different amounts on the different planets. This is because the gravitational field on their surface is different one from the other.

If the gravitational field was double that on Earth you would weigh twice as much as you do on Earth – and so would everything else!

Planet	Mass (Earth = 1000)	Radius (km)	Density (kg/m3	Gravitational field (N/kg)	Mass/Radius2 (N/kg)
Mercury	55	2440	5420	3.8	9.2
Venus	812	6050	5250	8.8	22.2
Earth	1000	6400	5510	9.8	24.4
Mars	110	3380	3960	3.8	9.6
Jupiter	317000	71400	1330	25	62.2
Saturn	95000	60400	680	10.4	26.0
Uranus	14500	23600	1600	10.4	26.0
Neptune	16700	22300	1650	13.8	33.6

You should be able to see from the table that this gravitational field at the surface of a planet does not just depend on the mass of the planet. For example, if you look at the gravitational field at the surface of Saturn it is the same as that on the surface of Uranus although Saturn is much more massive.

The size of this gravitational field is very important – if it too large we would be pulled so strongly to the surface that we would be crushed. For this reason creatures that lived on planets with high gravitational fields (high g) would need strong skeletons and really thick legs!

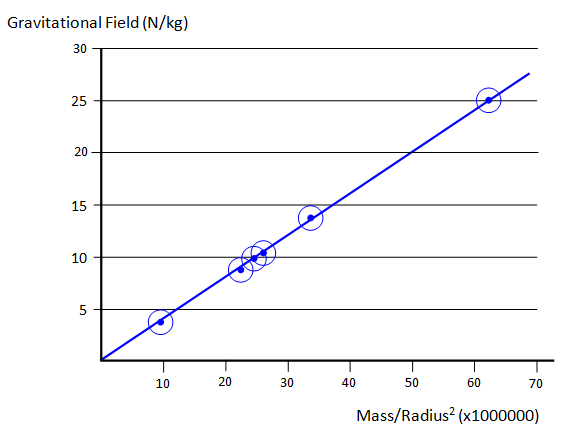
The graph shows the way in which mass and radius affect the size of the gravitational field.

The following graph gives you a way of working out the gravitational field on any planet, or other astronomical body such as one of the moons in the solar system, if you know its mass and its radius.

Pluto (a minor planet orbiting the Sun usually outside the orbit of Neptune) has a mass of 2.1 (compared with the Earth at 1000) and a mean radius of 1195 km. From a detailed version of the graph the graph the value of g on the surface of Pluto would be 0.58 N/kg.

Another way of finding the value of g is to use the radius of the planet and its density.


Use the data four the first four planets in the table to plot a graph of the surface gravitational field (g) against the (density x radius)/1000000.

Use your graph to work out the surface gravitational field on the following moons of the Solar System.


(Photograph: courtesy of NASA)