
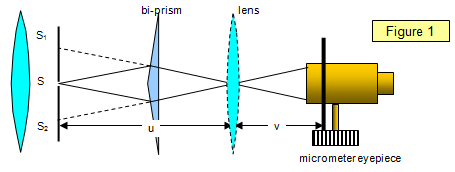
An alternative method to the classic Young's slits experiment for measuring the wavelength of light is that due to Fresnel. The apparatus
is shown in the following diagram (Figure 1).
Monochromatic light from a narrow slit S falls
on the bi-prism, the axis of which must be in line with the slit. The refracting angles of the bi-
prism are very small, usually about 0.25o. This prism forms two virtual images of the
slit S1 and S2 in the plane of S, and these two virtual images act
as the sources for two sets of waves which overlap and produce an interference pattern on the
screen.
The fringes are much brighter than those produced by Young's slits, because of the
very much greater amount of light that can pass through the prism compared with that passing
through the double slit arrangement.
The formula used is the same as for Young's slits, the
only problem being the measurement of the separation of the two virtual sources
S1 and S2.
This can be done by placing a convex lens
between the bi-prism and the screen or eyepiece and measuring the separation (s) of the
images of S1 and S2 produced by the lens. If the object and
image distances (u and v) are found, the value of d can be calculated from